It was 20 years ago that Barron’s published Amazon.bomb, an article calling into question Amazon’s business model during its infancy. Reading it now seems laughable. For example, take this passage:
Unfortunately for Bezos, Amazon is now entering a stage in which investors will be less willing to rely on his charisma and more demanding of answers to tough questions like, when will this company actually turn a profit? And how will Amazon triumph over a slew of new competitors who have deep pockets and new technologies?
We tried to ask Bezos, but he declined to make himself or any other executives of the company available. He can ignore Barron’s, but he can’t ignore the questions.
The passage is funny because we have the benefit of hindsight. We know how the story ends. As Barry Ritholtz noted in his recent post about this article:
The total gains since the Amazon.bomb column are about 4,606%, and an annualized average return of 21.1% – about quadruple what the S&P500 (with dividends) compounded – 202% total or 5.67% annualized – over the same period of time.
It’s easy to see this statistic and make fun of the Amazon.bomb author for getting it so wrong over the long run. But, that’s the simplistic takeaway from the article. That’s first-order thinking.
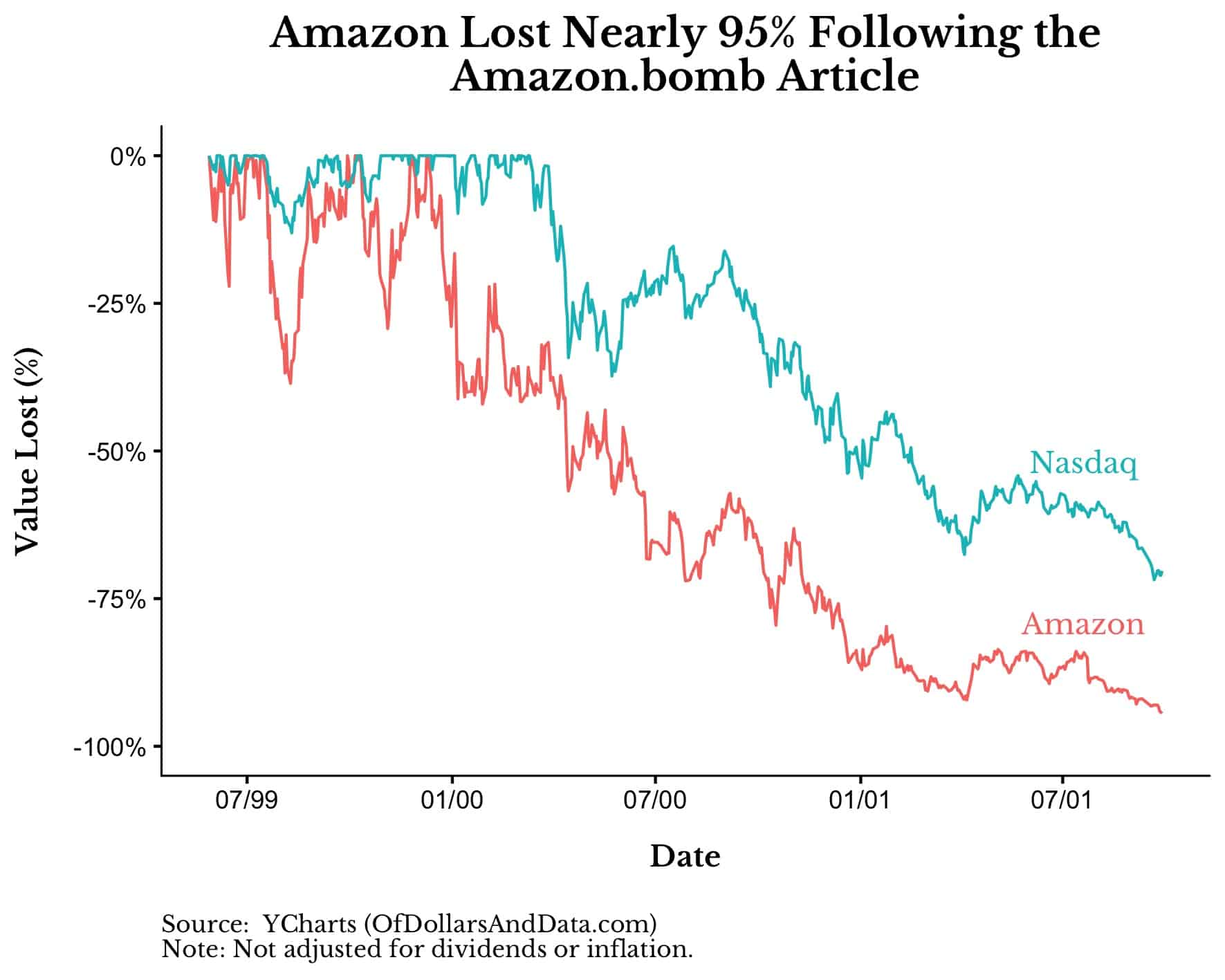
What you really need to do is look at how Amazon performed immediately following the article’s publication instead of over the next two decades. In this respect, as Barry noted, the article was spot on. In the 28 months following the publication of Amazon.bomb, Amazon’s stock declined by nearly 95%:
Relative to the NASDAQ Composite, which was down 70% over this same time period, Amazon declined an additional 83%. Even if you don’t fully agree with Amazon.bomb, you have to admit that the author’s analysis explains some of the additional 83% decline that Amazon experienced.
[Technical note: Amazon’s additional decline below the NASDAQ is not 25% because percentages are not additive. If you are down 70%, you would need to lose an additional 83% of your remaining capital to have a total loss of 95%. Mathematically: (1 – 0.7) * (1 – 0.83) = (1 – 0.95). Thank you for coming to my TED talk.]
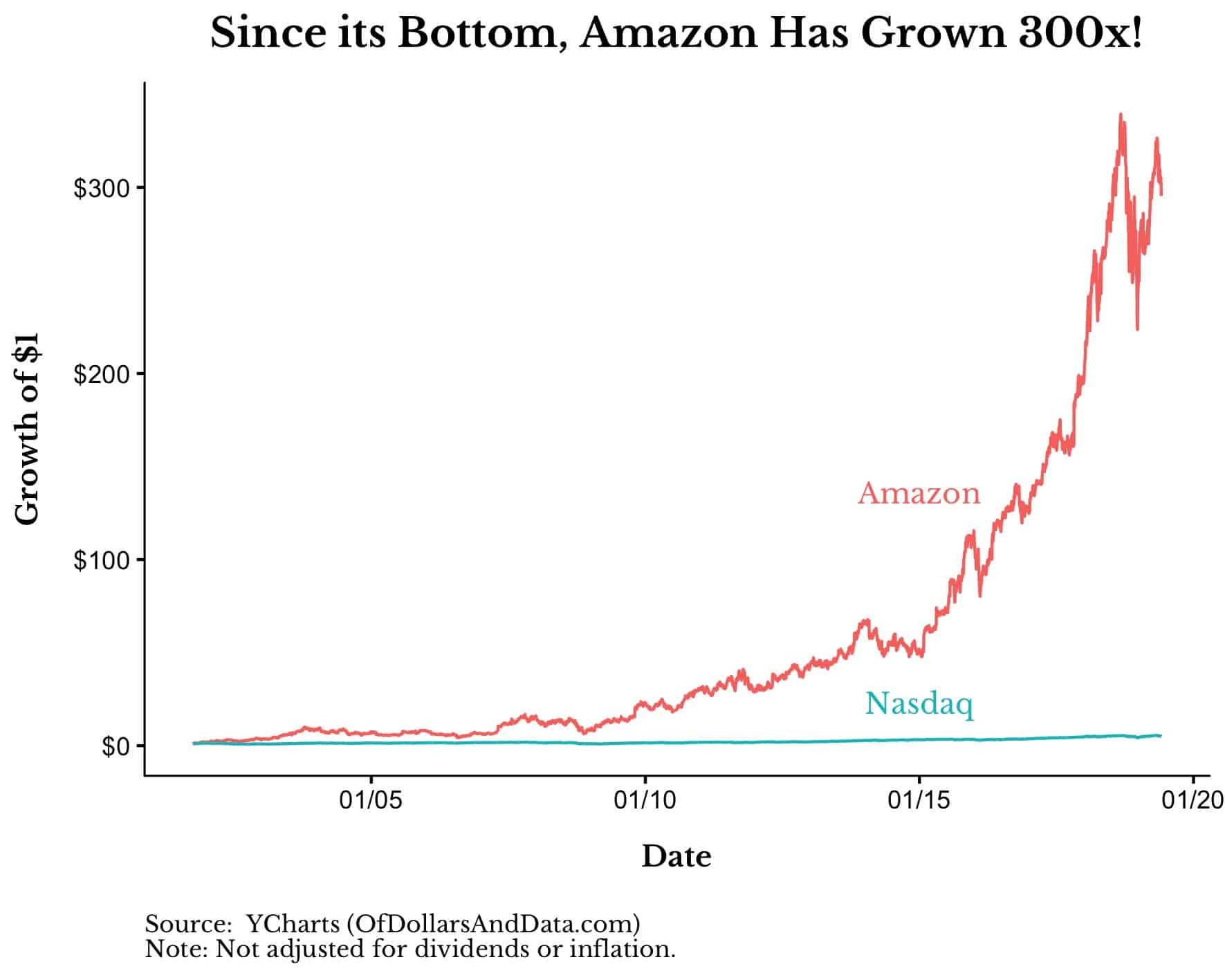
But, this is what makes investing so hard. Someone who read Amazon.bomb may have been convinced that the risk of holding Amazon wasn’t worth it. And they would have been vindicated when Amazon hit bottom on September 28, 2001. But, then this happened:
From Amazon’s DotCom bottom, an investment of $1 would have grown to $300 compared to only $5 if it had been invested in the NASDAQ. Of course, no investor could have known this at the time. No one could have foreseen all of the things Amazon would go on to do (i.e. Prime, AWS, etc.) to create that magnificent growth.
This is what makes investing so tough. You can be rewarded in the short run for things that don’t matter in the long run, and you can be punished in the short run for things that do matter in the long run. You can be wrong even when you get it right.
The investment world is littered with people who were wrong when they appeared to be right and vice versa. One of my favorite examples is Michael Burry, the investor who foresaw the housing bubble as early as 2005, but took losses on his portfolio for years while he waited for it to burst. Burry tells of the difficulties of being wrong when he had it right in The Big Short:
The data from the mortgage services was worse every month—the loans underlying the bonds were going bad at faster rates—and yet the price of insuring those loans, they said was falling. “Logic had failed me,” Burry said. “I couldn’t explain the outcome I was seeing.”
Burry, like others shorting mortgages in 2005-2007, watched dumbfounded as mortgage-backed securities prices rose while the underlying fundamentals deteriorated. Though their conclusion was accurate, the market did not immediately agree with them. As the famous phrase goes:
The market can stay irrational longer than you can stay solvent.
This is why shorting and market timing are so difficult. Because there is no iron law of investing that states that fundamentals have to matter. Yes, Ben Graham once said, “In the short run the market is a voting machine, but in the long run it is a weighing machine,” but just how long is the long run? Whatever the market says it is.
For example, if a group of investors with sufficient money want to pay 50 times earnings for a stock, then that’s what the stock will sell for. As I have written previously:
Assets are not priced based upon fundamentals, but by what someone else is willing to pay for them.
And no one is immune from this. Not even Warren Buffett. His multiple periods of underperformance demonstrate that even the greats will look like they got it wrong even when they are correct. Because superior investing isn’t just about being right, but being right and having the market agree with you…eventually.
Why Being “Right” is Overrated
While being right is great for an investor, what’s even better is not having to be right. Yes, those investors who can generate superior returns will outperform those who cannot, but at what cost and with what risk? I prefer taking the route that requires the least number of assumptions for me to have the highest probability of growing my wealth.
You know what investment assumptions have to be true for me to grow wealthy?
- My risky assets (i.e. global stocks, real estate) have to return 4-6% above my safe assets (i.e. bonds) after inflation for the next few decades
That’s it. I don’t need to be a contrarian. I don’t need to do any DCFs. I don’t need to know the future. As a passive investor, I just need some positive risk premia and the rest will take care of itself.
This is why being right is overrated, because not only do active investors require having to know more than the market, but they also need a positive risk premia as well. As I have stated before, “Don’t pray for alpha, pray for beta.” Happy investing and thank you for reading!
If you liked this post, consider signing up for my newsletter.
This is post 128. Any code I have related to this post can be found here with the same numbering: https://github.com/nmaggiulli/of-dollars-and-data